Line K Lies in the Xy Plane
Y 2x and the hyperbolas xy 1 xy 2. Thus for 3-dimensional spaces one needs to show that 1 every point lies in 3 distinct planes 2 every two planes intersect in a unique line and a dual version of 3 to the effect.

Distance Formula Distance Formula Between Two Points In X Y Plane Distance Formula Formula Science Quiz
But u T u u u and u T x u x so c u x u u is a.
. Special forms of the equation of a plane. Sketch the region S in the uv-plane corresponding to R. In addition we will define the gradient vector to help with some of the notation and work here.
In the section we introduce the concept of directional derivatives. If the intersection of plane P and Q is coplanar with the intersection of plane R and S then so are the respective intersections of planes P and R Q and S. 1 Intercept form of the equation of a plane.
Here mathbfrxyz is a vector representing any general point on the line. Figure 1334 page 759. The intercept form of the equation of a plane is where a b and c are the x y and z intercepts respectively all intercepts assumed to be non-zero.
The word is derived from the Latin words quadri a variant of four and latus meaning sideAnother name for it is tetragon derived from greek tetra meaning four and gon meaning corner or angle in analogy to eg pentagon. The part in the first quadrant can be rewritten as v u and v 2u respectively. With directional derivatives we can now ask how a function is changing if we allow all the independent variables to change rather than holding all but one constant as we had to do with partial derivatives.
Mathbfr_0x_0y_0z_0 is a given point that lies on the line. Example Orthogonal projection onto a line Let L Span u be a line in R n and let x be a vector in R n. The lines y x and y 2x in the xy-plane correspond to v uv v 2uv in the uv-plane respectively.
Gon being angle also is at the root of calling. Restricted to a plane. Mathbfnn_xn_yn_z is a given unit vector that has a magnitude of unity that is parallel to the line.
The equation z k represents a plane parallel to the xy plane and k units from it. Since rr C or more explicitly rt rt C where C is a constant then we see that the position vector r is always orthogonal to vector C. Therefore r in standard position lies in a plane with C as its normal vector and mass m is in this plane for all values of t.
In geometry a quadrilateral is a four-sided polygon having four edges sides and four corners vertices. By the theorem to find x L we must solve the matrix equation u T uc u T x where we regard u as an n 1 matrix the column space of this matrix is exactly L.

In The Xy Plane Above Line L Is Parallel To Line K What Is The Value Of P

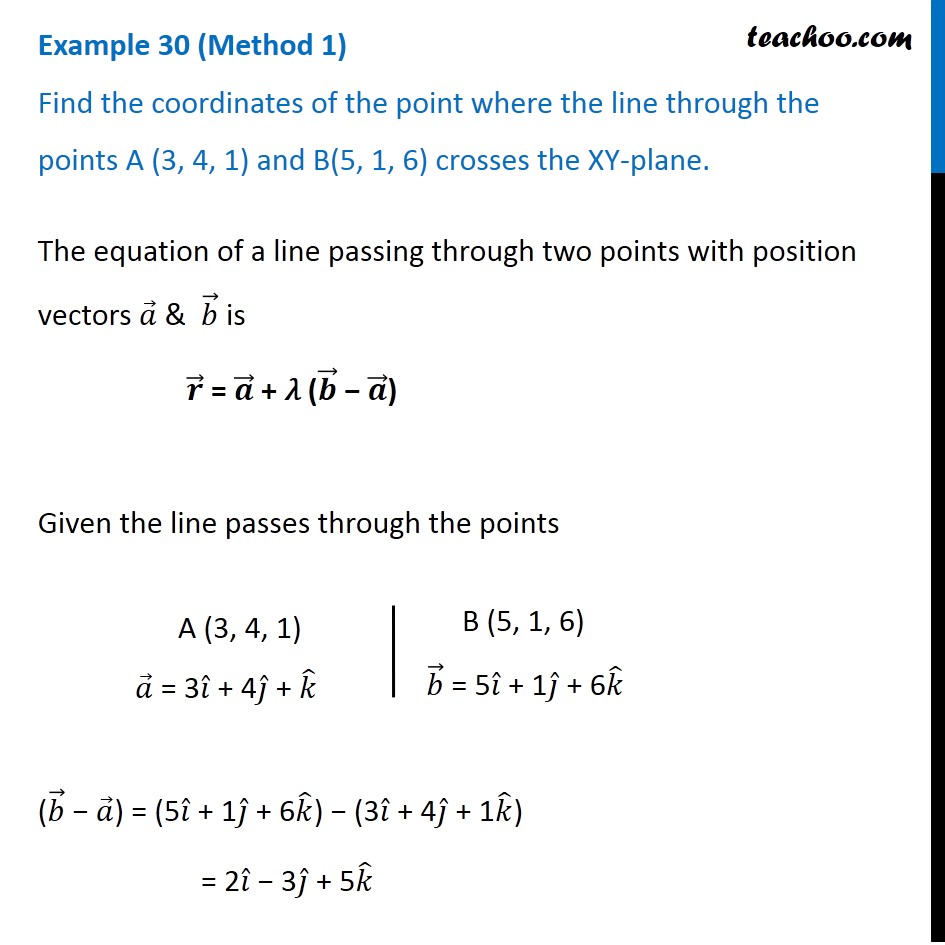
Example 30 Find Coordinates Of Point Where Line Through A 3 4 1

A Line In The Xy Plane Passes Through The Origin And Has A Slope Of 1 7 Which Of The Following Youtube

Find The Coordinates Of A Point Where Line Intersects Xy Plane R3 Vector Youtube
No comments for "Line K Lies in the Xy Plane"
Post a Comment